Sensitivity to ISO

using ModelingToolkit
using OrdinaryDiffEq, SteadyStateDiffEq, DiffEqCallbacks
using Plots
using LsqFit
using CaMKIIModel
using CaMKIIModel: μM, hil, Hz, hilr, second
Plots.default(lw=1.5)

Setup b1AR system

@parameters ATP = 5000μM ISO = 0μM
sys = get_bar_sys(ATP, ISO; simplify=true)

\[\begin{split} \begin{align} \frac{\mathrm{d} \mathtt{RyRp}\left( t \right)}{\mathrm{d}t} &= \frac{ - \mathtt{PP1\_RyR} \mathtt{epsilon} \mathtt{kcat\_pp1\_RyR} \mathtt{RyRp}\left( t \right)}{\mathtt{Km\_pp1\_RyR} + \mathtt{epsilon} \mathtt{RyRp}\left( t \right)} + \frac{\mathtt{PKAII\_RyRtot} \mathtt{epsilon} \mathtt{kcat\_pka\_RyR} \mathtt{PKACII}\left( t \right) \mathtt{RyRn}\left( t \right)}{\mathtt{PKAIItot} \left( \mathtt{Km\_pka\_RyR} + \mathtt{epsilon} \mathtt{RyRn}\left( t \right) \right)} + \frac{ - \mathtt{PP2A\_RyR} \mathtt{epsilon} \mathtt{kcat\_pp2a\_RyR} \mathtt{RyRp}\left( t \right)}{\mathtt{Km\_pp2a\_RyR} + \mathtt{epsilon} \mathtt{RyRp}\left( t \right)} \\ \frac{\mathrm{d} \mathtt{KURp}\left( t \right)}{\mathrm{d}t} &= \frac{ - \mathtt{PP1\_KURtot} \mathtt{epsilon} \mathtt{k\_pp1\_KUR} \mathtt{KURp}\left( t \right)}{\mathtt{Km\_pp1\_KUR} + \mathtt{epsilon} \mathtt{KURp}\left( t \right)} + \frac{\mathtt{PKAII\_KURtot} \mathtt{epsilon} \mathtt{k\_pka\_KUR} \mathtt{KURn}\left( t \right) \mathtt{PKACII}\left( t \right)}{\mathtt{PKAIItot} \left( \mathtt{Km\_pka\_KUR} + \mathtt{epsilon} \mathtt{KURn}\left( t \right) \right)} \\ \frac{\mathrm{d} \mathtt{LCCbp}\left( t \right)}{\mathrm{d}t} &= \frac{\mathtt{PKACII\_LCCtotBA} \mathtt{epsilon} \mathtt{k\_PKA\_LCC} \mathtt{LCCb}\left( t \right) \mathtt{PKACII}\left( t \right)}{\mathtt{PKAIItot} \left( \mathtt{Km\_PKA\_LCC} + \mathtt{epsilon} \mathtt{LCCb}\left( t \right) \right)} + \frac{ - \mathtt{PP1\_LCC} \mathtt{epsilon} \mathtt{k\_PP1\_LCC} \mathtt{LCCbp}\left( t \right)}{\mathtt{Km\_PP1\_LCC} + \mathtt{epsilon} \mathtt{LCCbp}\left( t \right)} \\ \frac{\mathrm{d} \mathtt{LCCap}\left( t \right)}{\mathrm{d}t} &= \frac{\mathtt{PKACII\_LCCtotBA} \mathtt{epsilon} \mathtt{k\_PKA\_LCC} \mathtt{PKACII}\left( t \right) \mathtt{LCCa}\left( t \right)}{\mathtt{PKAIItot} \left( \mathtt{Km\_PKA\_LCC} + \mathtt{epsilon} \mathtt{LCCa}\left( t \right) \right)} + \frac{ - \mathtt{PP2A\_LCC} \mathtt{epsilon} \mathtt{k\_PP2A\_LCC} \mathtt{LCCap}\left( t \right)}{\mathtt{Km\_PP2A\_LCC} + \mathtt{epsilon} \mathtt{LCCap}\left( t \right)} \\ \frac{\mathrm{d} \mathtt{TnIp}\left( t \right)}{\mathrm{d}t} &= \frac{ - \mathtt{PP2A\_TnI} \mathtt{k\_PP2A\_TnI} \mathtt{TnIp}\left( t \right)}{\mathtt{Km\_PP2A\_TnI} + \mathtt{TnIp}\left( t \right)} + \frac{\mathtt{k\_PKA\_TnI} \mathtt{PKACI}\left( t \right) \mathtt{TnI}\left( t \right)}{\mathtt{Km\_PKA\_TnI} + \mathtt{TnI}\left( t \right)} \\ \frac{\mathrm{d} \mathtt{PLMp}\left( t \right)}{\mathrm{d}t} &= \frac{\mathtt{k\_PKA\_PLM} \mathtt{PKACI}\left( t \right) \mathtt{PLM}\left( t \right)}{\mathtt{Km\_PKA\_PLM} + \mathtt{PLM}\left( t \right)} + \frac{ - \mathtt{k\_PP1\_PLM} \mathtt{PP1}\left( t \right) \mathtt{PLMp}\left( t \right)}{\mathtt{Km\_PP1\_PLM} + \mathtt{PLMp}\left( t \right)} \\ \frac{\mathrm{d} \mathtt{PLBp}\left( t \right)}{\mathrm{d}t} &= \frac{ - \mathtt{k\_PP1\_PLB} \mathtt{PP1}\left( t \right) \mathtt{PLBp}\left( t \right)}{\mathtt{Km\_PP1\_PLB} + \mathtt{PLBp}\left( t \right)} + \frac{\mathtt{k\_PKA\_PLB} \mathtt{PLB}\left( t \right) \mathtt{PKACI}\left( t \right)}{\mathtt{Km\_PKA\_PLB} + \mathtt{PLB}\left( t \right)} \\ \frac{\mathrm{d} \mathtt{I1p\_PP1}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kr\_PP1\_I1} \mathtt{I1p\_PP1}\left( t \right) + \mathtt{kf\_PP1\_I1} \mathtt{PP1}\left( t \right) \mathtt{I1p}\left( t \right) \\ \frac{\mathrm{d} \mathtt{I1p}\left( t \right)}{\mathrm{d}t} &= \frac{\mathtt{k\_PKA\_I1} \mathtt{PKACI}\left( t \right) \mathtt{I1}\left( t \right)}{\mathtt{Km\_PKA\_I1} + \mathtt{I1}\left( t \right)} + \frac{ - \mathtt{Vmax\_PP2A\_I1} \mathtt{I1p}\left( t \right)}{\mathtt{Km\_PP2A\_I1} + \mathtt{I1p}\left( t \right)} + \mathtt{kr\_PP1\_I1} \mathtt{I1p\_PP1}\left( t \right) - \mathtt{kf\_PP1\_I1} \mathtt{PP1}\left( t \right) \mathtt{I1p}\left( t \right) \\ \frac{\mathrm{d} \mathtt{PKACII\_PKI}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kr\_PKA\_PKI} \mathtt{PKACII\_PKI}\left( t \right) + \mathtt{kf\_PKA\_PKI} \mathtt{PKI}\left( t \right) \mathtt{PKACII}\left( t \right) \\ \frac{\mathrm{d} \mathtt{PKACI\_PKI}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kr\_PKA\_PKI} \mathtt{PKACI\_PKI}\left( t \right) + \mathtt{kf\_PKA\_PKI} \mathtt{PKACI}\left( t \right) \mathtt{PKI}\left( t \right) \\ \frac{\mathrm{d} \mathtt{PKACII}\left( t \right)}{\mathrm{d}t} &= \mathtt{kf\_RcAMPcAMP\_C} \mathtt{RCcAMPcAMP\_II}\left( t \right) + \mathtt{kr\_PKA\_PKI} \mathtt{PKACII\_PKI}\left( t \right) - \mathtt{kf\_PKA\_PKI} \mathtt{PKI}\left( t \right) \mathtt{PKACII}\left( t \right) - \mathtt{kr\_RcAMPcAMP\_C} \mathtt{PKACII}\left( t \right) \mathtt{RcAMPcAMP\_II}\left( t \right) \\ \frac{\mathrm{d} \mathtt{PKACI}\left( t \right)}{\mathrm{d}t} &= \mathtt{kf\_RcAMPcAMP\_C} \mathtt{RCcAMPcAMP\_I}\left( t \right) + \mathtt{kr\_PKA\_PKI} \mathtt{PKACI\_PKI}\left( t \right) - \mathtt{kf\_PKA\_PKI} \mathtt{PKACI}\left( t \right) \mathtt{PKI}\left( t \right) - \mathtt{kr\_RcAMPcAMP\_C} \mathtt{PKACI}\left( t \right) \mathtt{RcAMPcAMP\_I}\left( t \right) \\ \frac{\mathrm{d} \mathtt{RCcAMPcAMP\_II}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kf\_RcAMPcAMP\_C} \mathtt{RCcAMPcAMP\_II}\left( t \right) - \mathtt{kr\_RCcAMP\_cAMP} \mathtt{RCcAMPcAMP\_II}\left( t \right) + \mathtt{kf\_RCcAMP\_cAMP} \mathtt{RCcAMP\_II}\left( t \right) \mathtt{cAMP}\left( t \right) + \mathtt{kr\_RcAMPcAMP\_C} \mathtt{PKACII}\left( t \right) \mathtt{RcAMPcAMP\_II}\left( t \right) \\ \frac{\mathrm{d} \mathtt{RCcAMPcAMP\_I}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kf\_RcAMPcAMP\_C} \mathtt{RCcAMPcAMP\_I}\left( t \right) - \mathtt{kr\_RCcAMP\_cAMP} \mathtt{RCcAMPcAMP\_I}\left( t \right) + \mathtt{kf\_RCcAMP\_cAMP} \mathtt{RCcAMP\_I}\left( t \right) \mathtt{cAMP}\left( t \right) + \mathtt{kr\_RcAMPcAMP\_C} \mathtt{PKACI}\left( t \right) \mathtt{RcAMPcAMP\_I}\left( t \right) \\ \frac{\mathrm{d} \mathtt{RCcAMP\_II}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kr\_RC\_cAMP} \mathtt{RCcAMP\_II}\left( t \right) + \mathtt{kr\_RCcAMP\_cAMP} \mathtt{RCcAMPcAMP\_II}\left( t \right) + \mathtt{kf\_RC\_cAMP} \mathtt{RC\_II}\left( t \right) \mathtt{cAMP}\left( t \right) - \mathtt{kf\_RCcAMP\_cAMP} \mathtt{RCcAMP\_II}\left( t \right) \mathtt{cAMP}\left( t \right) \\ \frac{\mathrm{d} \mathtt{RCcAMP\_I}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kr\_RC\_cAMP} \mathtt{RCcAMP\_I}\left( t \right) + \mathtt{kr\_RCcAMP\_cAMP} \mathtt{RCcAMPcAMP\_I}\left( t \right) + \mathtt{kf\_RC\_cAMP} \mathtt{RC\_I}\left( t \right) \mathtt{cAMP}\left( t \right) - \mathtt{kf\_RCcAMP\_cAMP} \mathtt{RCcAMP\_I}\left( t \right) \mathtt{cAMP}\left( t \right) \\ \frac{\mathrm{d} \mathtt{cAMP}\left( t \right)}{\mathrm{d}t} &= \frac{\mathtt{ATP} \mathtt{k\_AC\_basal} \mathtt{AC}\left( t \right)}{\mathtt{ATP} + \mathtt{Km\_AC\_basal}} + \frac{\mathtt{ATP} \mathtt{k\_AC\_Gsa} \mathtt{AC\_GsaGTP}\left( t \right)}{\mathtt{ATP} + \mathtt{Km\_AC\_Gsa}} + \frac{\left( - \mathtt{k\_cAMP\_PDE} \mathtt{PDE}\left( t \right) - \mathtt{k\_cAMP\_PDEp} \mathtt{PDEp}\left( t \right) \right) \mathtt{cAMP}\left( t \right)}{\mathtt{Km\_PDE\_cAMP} + \mathtt{cAMP}\left( t \right)} + \mathtt{kr\_RC\_cAMP} \mathtt{RCcAMP\_I}\left( t \right) + \mathtt{kr\_RC\_cAMP} \mathtt{RCcAMP\_II}\left( t \right) + \mathtt{kr\_RCcAMP\_cAMP} \mathtt{RCcAMPcAMP\_II}\left( t \right) + \mathtt{kr\_RCcAMP\_cAMP} \mathtt{RCcAMPcAMP\_I}\left( t \right) - \mathtt{kf\_RC\_cAMP} \mathtt{RC\_II}\left( t \right) \mathtt{cAMP}\left( t \right) - \mathtt{kf\_RC\_cAMP} \mathtt{RC\_I}\left( t \right) \mathtt{cAMP}\left( t \right) - \mathtt{kf\_RCcAMP\_cAMP} \mathtt{RCcAMP\_I}\left( t \right) \mathtt{cAMP}\left( t \right) - \mathtt{kf\_RCcAMP\_cAMP} \mathtt{RCcAMP\_II}\left( t \right) \mathtt{cAMP}\left( t \right) \\ \frac{\mathrm{d} \mathtt{PDEp}\left( t \right)}{\mathrm{d}t} &= - \mathtt{k\_PP\_PDE} \mathtt{PDEp}\left( t \right) + \mathtt{k\_PKA\_PDE} \mathtt{PDE}\left( t \right) \mathtt{PKACII}\left( t \right) \\ \frac{\mathrm{d} \mathtt{AC\_GsaGTP}\left( t \right)}{\mathrm{d}t} &= - \mathtt{k\_G\_hyd} \mathtt{AC\_GsaGTP}\left( t \right) - \mathtt{kr\_AC\_Gsa} \mathtt{AC\_GsaGTP}\left( t \right) + \mathtt{kf\_AC\_Gsa} \mathtt{AC}\left( t \right) \mathtt{GsaGTP}\left( t \right) \\ \frac{\mathrm{d} \mathtt{b1AR\_S301}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kr\_PKA} \mathtt{b1AR\_S301}\left( t \right) + \mathtt{kf\_PKA} \mathtt{PKACI}\left( t \right) \mathtt{LR}\left( t \right) + \mathtt{kf\_PKA} \mathtt{PKACI}\left( t \right) \mathtt{LRG}\left( t \right) + \mathtt{kf\_PKA} \mathtt{PKACI}\left( t \right) \mathtt{b1AR}\left( t \right) \\ \frac{\mathrm{d} \mathtt{b1AR\_S464}\left( t \right)}{\mathrm{d}t} &= \mathtt{kf\_bARK} \mathtt{LR}\left( t \right) + \mathtt{kf\_bARK} \mathtt{LRG}\left( t \right) - \mathtt{kr\_bARK} \mathtt{b1AR\_S464}\left( t \right) \\ \frac{\mathrm{d} \mathtt{GsaGDP}\left( t \right)}{\mathrm{d}t} &= \mathtt{k\_G\_hyd} \mathtt{AC\_GsaGTP}\left( t \right) + \mathtt{k\_G\_hyd} \mathtt{GsaGTP}\left( t \right) - \mathtt{k\_G\_reassoc} \mathtt{Gsby}\left( t \right) \mathtt{GsaGDP}\left( t \right) \\ \frac{\mathrm{d} \mathtt{GsaGTP}\left( t \right)}{\mathrm{d}t} &= \mathtt{k\_G\_act} \mathtt{RG}\left( t \right) + \mathtt{k\_G\_act} \mathtt{LRG}\left( t \right) - \mathtt{k\_G\_hyd} \mathtt{GsaGTP}\left( t \right) + \mathtt{kr\_AC\_Gsa} \mathtt{AC\_GsaGTP}\left( t \right) - \mathtt{kf\_AC\_Gsa} \mathtt{AC}\left( t \right) \mathtt{GsaGTP}\left( t \right) \\ \frac{\mathrm{d} \mathtt{RG}\left( t \right)}{\mathrm{d}t} &= - \mathtt{k\_G\_act} \mathtt{RG}\left( t \right) - \mathtt{kr\_RG} \mathtt{RG}\left( t \right) + \mathtt{kf\_RG} \mathtt{Gs}\left( t \right) \mathtt{b1AR}\left( t \right) \\ \frac{\mathrm{d} \mathtt{LRG}\left( t \right)}{\mathrm{d}t} &= - \mathtt{k\_G\_act} \mathtt{LRG}\left( t \right) - \mathtt{kf\_bARK} \mathtt{LRG}\left( t \right) - \mathtt{kr\_LRG} \mathtt{LRG}\left( t \right) + \mathtt{kf\_LRG} \mathtt{LR}\left( t \right) \mathtt{Gs}\left( t \right) - \mathtt{kf\_PKA} \mathtt{PKACI}\left( t \right) \mathtt{LRG}\left( t \right) \\ \frac{\mathrm{d} \mathtt{LR}\left( t \right)}{\mathrm{d}t} &= - \mathtt{kf\_bARK} \mathtt{LR}\left( t \right) - \mathtt{kr\_LR} \mathtt{LR}\left( t \right) + \mathtt{kr\_LRG} \mathtt{LRG}\left( t \right) + \mathtt{kr\_bARK} \mathtt{b1AR\_S464}\left( t \right) + \mathtt{ISO} \mathtt{kf\_LR} \mathtt{b1AR}\left( t \right) - \mathtt{kf\_LRG} \mathtt{LR}\left( t \right) \mathtt{Gs}\left( t \right) - \mathtt{kf\_PKA} \mathtt{PKACI}\left( t \right) \mathtt{LR}\left( t \right) \end{align} \end{split}\]

prob = SteadyStateProblem(sys, [])
alg = DynamicSS(Rodas5P())

SteadyStateDiffEq.DynamicSS{OrdinaryDiffEqRosenbrock.Rodas5P{0, ADTypes.AutoForwardDiff{nothing, Nothing}, Nothing, typeof(OrdinaryDiffEqCore.DEFAULT_PRECS), Val{:forward}(), true, nothing, typeof(OrdinaryDiffEqCore.trivial_limiter!), typeof(OrdinaryDiffEqCore.trivial_limiter!)}, Float64}(OrdinaryDiffEqRosenbrock.Rodas5P{0, ADTypes.AutoForwardDiff{nothing, Nothing}, Nothing, typeof(OrdinaryDiffEqCore.DEFAULT_PRECS), Val{:forward}(), true, nothing, typeof(OrdinaryDiffEqCore.trivial_limiter!), typeof(OrdinaryDiffEqCore.trivial_limiter!)}(nothing, OrdinaryDiffEqCore.DEFAULT_PRECS, OrdinaryDiffEqCore.trivial_limiter!, OrdinaryDiffEqCore.trivial_limiter!, ADTypes.AutoForwardDiff()), Inf)

Log scale for ISO concentration

iso = exp10.(range(log10(1e-4μM), log10(1μM), length=1001))

1001-element Vector{Float64}:
 0.0001
 0.00010092528860766844
 0.00010185913880541169
 0.00010280162981264735
 0.00010375284158180127
 0.00010471285480508996
 0.00010568175092136585
 0.0001066596121230258
 0.0001076465213629835
 0.00010864256236170655
 ⋮
 0.9289663867799364
 0.9375620069258802
 0.9462371613657931
 0.954992586021436
 0.9638290236239705
 0.9727472237769651
 0.9817479430199844
 0.9908319448927676
 1.0

prob_func = (prob, i, repeat) -> remake(prob, p=[ISO => iso[i]])
trajectories = length(iso)
sol = solve(prob, alg; abstol=1e-10, reltol=1e-10) ## warmup
sim = solve(EnsembleProblem(prob; prob_func, safetycopy=false), alg; trajectories, abstol=1e-10, reltol=1e-10)

EnsembleSolution Solution of length 1001 with uType:
SciMLBase.NonlinearSolution{Float64, 1, Vector{Float64}, Vector{Float64}, SciMLBase.SteadyStateProblem{Vector{Float64}, true, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}}, SteadyStateDiffEq.DynamicSS{OrdinaryDiffEqRosenbrock.Rodas5P{0, ADTypes.AutoForwardDiff{nothing, ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}}, Nothing, typeof(OrdinaryDiffEqCore.DEFAULT_PRECS), Val{:forward}(), true, nothing, typeof(OrdinaryDiffEqCore.trivial_limiter!), typeof(OrdinaryDiffEqCore.trivial_limiter!)}, Float64}, SciMLBase.ODESolution{Float64, 2, Vector{Vector{Float64}}, Nothing, Nothing, Vector{Float64}, Vector{Vector{Vector{Float64}}}, Nothing, SciMLBase.ODEProblem{Vector{Float64}, Tuple{Float64, Float64}, true, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardODEProblem}, OrdinaryDiffEqRosenbrock.Rodas5P{0, ADTypes.AutoForwardDiff{nothing, ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}}, Nothing, typeof(OrdinaryDiffEqCore.DEFAULT_PRECS), Val{:forward}(), true, nothing, typeof(OrdinaryDiffEqCore.trivial_limiter!), typeof(OrdinaryDiffEqCore.trivial_limiter!)}, OrdinaryDiffEqCore.InterpolationData{SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Vector{Vector{Float64}}, Vector{Float64}, Vector{Vector{Vector{Float64}}}, Nothing, OrdinaryDiffEqRosenbrock.RosenbrockCache{Vector{Float64}, Vector{Float64}, Float64, Vector{Float64}, Matrix{Float64}, Matrix{Float64}, OrdinaryDiffEqRosenbrock.RodasTableau{Float64, Float64}, SciMLBase.TimeGradientWrapper{true, SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Vector{Float64}, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}}, SciMLBase.UJacobianWrapper{true, SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Float64, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}}, LinearSolve.LinearCache{Matrix{Float64}, Vector{Float64}, Vector{Float64}, SciMLBase.NullParameters, LinearSolve.DefaultLinearSolver, LinearSolve.DefaultLinearSolverInit{LinearAlgebra.LU{Float64, Matrix{Float64}, Vector{Int64}}, LinearAlgebra.QRCompactWY{Float64, Matrix{Float64}, Matrix{Float64}}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Tuple{LinearAlgebra.LU{Float64, Matrix{Float64}, Vector{Int64}}, Vector{Int64}}, Tuple{LinearAlgebra.LU{Float64, Matrix{Float64}, Vector{Int64}}, Vector{Int64}}, Nothing, Nothing, Nothing, LinearAlgebra.SVD{Float64, Float64, Matrix{Float64}, Vector{Float64}}, LinearAlgebra.Cholesky{Float64, Matrix{Float64}}, LinearAlgebra.Cholesky{Float64, Matrix{Float64}}, Tuple{LinearAlgebra.LU{Float64, Matrix{Float64}, Vector{Int32}}, Base.RefValue{Int32}}, Tuple{LinearAlgebra.LU{Float64, Matrix{Float64}, Vector{Int64}}, Base.RefValue{Int64}}, LinearAlgebra.QRPivoted{Float64, Matrix{Float64}, Vector{Float64}, Vector{Int64}}, Nothing, Nothing}, LinearSolve.InvPreconditioner{LinearAlgebra.Diagonal{Float64, Vector{Float64}}}, LinearAlgebra.Diagonal{Float64, Vector{Float64}}, Float64, Bool, LinearSolve.LinearSolveAdjoint{Missing}}, Tuple{DifferentiationInterfaceForwardDiffExt.ForwardDiffTwoArgJacobianPrep{Tuple{SciMLBase.UJacobianWrapper{true, SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Float64, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}}, Vector{Float64}, ADTypes.AutoForwardDiff{nothing, ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}}, Vector{Float64}, Tuple{}}, ForwardDiff.JacobianConfig{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 9, Tuple{Vector{ForwardDiff.Dual{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 9}}, Vector{ForwardDiff.Dual{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 9}}}}, Tuple{}}, DifferentiationInterfaceForwardDiffExt.ForwardDiffTwoArgJacobianPrep{Tuple{SciMLBase.UJacobianWrapper{true, SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Float64, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}}, Vector{Float64}, ADTypes.AutoForwardDiff{nothing, ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}}, Vector{Float64}, Tuple{}}, ForwardDiff.JacobianConfig{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 9, Tuple{Vector{ForwardDiff.Dual{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 9}}, Vector{ForwardDiff.Dual{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 9}}}}, Tuple{}}}, Tuple{DifferentiationInterfaceForwardDiffExt.ForwardDiffTwoArgDerivativePrep{Tuple{SciMLBase.TimeGradientWrapper{true, SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Vector{Float64}, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}}, Vector{Float64}, ADTypes.AutoForwardDiff{nothing, ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}}, Float64, Tuple{}}, ForwardDiff.DerivativeConfig{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Vector{ForwardDiff.Dual{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 1}}}, Tuple{}}, DifferentiationInterfaceForwardDiffExt.ForwardDiffTwoArgDerivativePrep{Tuple{SciMLBase.TimeGradientWrapper{true, SciMLBase.ODEFunction{true, true, SciMLBase.ODEFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9abf87c8, 0xbf21377d, 0x3cbaa2df, 0x5523dad7, 0xdd72ad22), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x32036410, 0xc20af136, 0x2e1afbba, 0x75aea465, 0x7c03e232), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, SciMLBase.OverrideInitData{SciMLBase.NonlinearProblem{Nothing, true, ModelingToolkit.MTKParameters{Vector{Float64}, StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}, SciMLBase.NonlinearFunction{true, SciMLBase.FullSpecialize, ModelingToolkit.GeneratedFunctionWrapper{(2, 2, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x9a1dc1ad, 0x71defabe, 0x857c130d, 0xdfc20b1f, 0x2cf6f9a1), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x00797acb, 0x0db5e856, 0xa0aff6bc, 0xf6b12faf, 0xd9a97c31), Nothing}}, LinearAlgebra.UniformScaling{Bool}, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, Nothing, ModelingToolkit.ObservedFunctionCache{ModelingToolkit.System}, Nothing, ModelingToolkit.System, Nothing, Nothing}, Base.Pairs{Symbol, Union{}, Tuple{}, @NamedTuple{}}, SciMLBase.StandardNonlinearProblem}, Nothing, Nothing, Nothing, ModelingToolkit.InitializationMetadata{ModelingToolkit.ReconstructInitializeprob{ModelingToolkit.var"#_getter#900"{Tuple{ComposedFunction{ModelingToolkit.PConstructorApplicator{typeof(identity)}, ModelingToolkit.ObservedWrapper{true, ModelingToolkit.GeneratedFunctionWrapper{(2, 3, true), RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0x42c03932, 0xaddf2ca6, 0x0a44b1ef, 0x4747ea09, 0x34bc7748), Nothing}, RuntimeGeneratedFunctions.RuntimeGeneratedFunction{(:ˍ₋out, :__mtk_arg_1, :___mtkparameters___, :t), ModelingToolkit.var"#_RGF_ModTag", ModelingToolkit.var"#_RGF_ModTag", (0xd2359f88, 0xfe2c3976, 0x012b459c, 0x7b3091e5, 0x1185196b), Nothing}}}}, Returns{StaticArraysCore.SizedVector{0, Float64, Vector{Float64}}}, Returns{Tuple{}}, Returns{Tuple{}}, Returns{Tuple{}}}}, ComposedFunction{typeof(identity), SymbolicIndexingInterface.MultipleGetters{SymbolicIndexingInterface.ContinuousTimeseries, Vector{Any}}}}, Nothing, ModelingToolkit.SetInitialUnknowns{SymbolicIndexingInterface.MultipleSetters{Vector{SymbolicIndexingInterface.ParameterHookWrapper{SymbolicIndexingInterface.SetParameterIndex{ModelingToolkit.ParameterIndex{SciMLStructures.Initials, Int64}}, SymbolicUtils.BasicSymbolic{Real}}}}}}, Val{true}}, Nothing}, Vector{Float64}, ModelingToolkit.MTKParameters{Vector{Float64}, Vector{Float64}, Tuple{}, Tuple{}, Tuple{}, Tuple{}}}, Vector{Float64}, ADTypes.AutoForwardDiff{nothing, ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}}, Float64, Tuple{}}, ForwardDiff.DerivativeConfig{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Vector{ForwardDiff.Dual{ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}, Float64, 1}}}, Tuple{}}}, Float64, OrdinaryDiffEqRosenbrock.Rodas5P{0, ADTypes.AutoForwardDiff{nothing, ForwardDiff.Tag{DiffEqBase.OrdinaryDiffEqTag, Float64}}, Nothing, typeof(OrdinaryDiffEqCore.DEFAULT_PRECS), Val{:forward}(), true, nothing, typeof(OrdinaryDiffEqCore.trivial_limiter!), typeof(OrdinaryDiffEqCore.trivial_limiter!)}, typeof(OrdinaryDiffEqCore.trivial_limiter!), typeof(OrdinaryDiffEqCore.trivial_limiter!)}, Nothing}, SciMLBase.DEStats, Nothing, Nothing, Nothing, Nothing}, Nothing, Nothing, Nothing}

"""Extract values from ensemble simulations by a symbol"""
extract(sim, k) = map(s -> s[k], sim)
"""Calculate Root Mean Square Error (RMSE)"""
rmse(fit) = sqrt(sum(abs2, fit.resid) / length(fit.resid))

Main.var"##230".rmse

xopts = (xlims=(iso[begin], iso[end]), minorgrid=true, xscale=:log10, xlabel="ISO (μM)",)
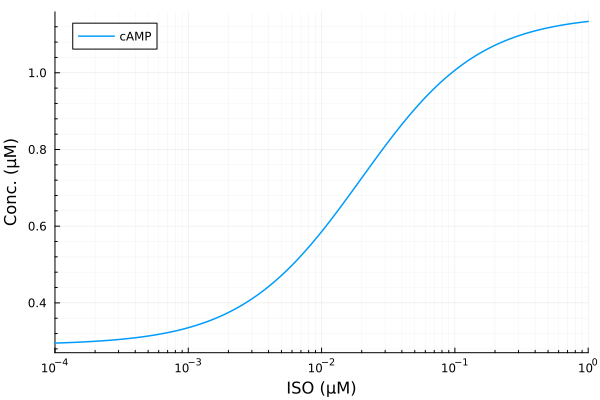
plot(iso, extract(sim, sys.cAMP); lab="cAMP", ylabel="Conc. (μM)", legend=:topleft, xopts...)

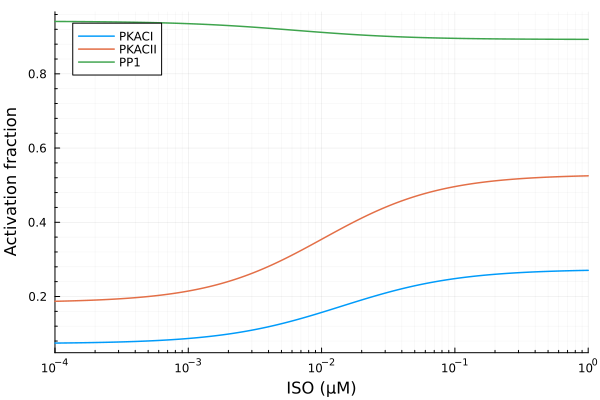
plot(iso, extract(sim, sys.PKACI / sys.RItot); lab="PKACI", ylabel="Activation fraction")
plot!(iso, extract(sim, sys.PKACII / sys.RIItot), lab="PKACII")
plot!(iso, extract(sim, sys.PP1 / sys.PP1totBA), lab="PP1", legend=:topleft; xopts...)

Fitting active PKACI

@. model(x, p) = p[1] * x / (x + p[2]) + p[3]
xdata = iso
ydata = extract(sim, sys.PKACI / sys.RItot)
p0 = [0.3, 0.01μM, 0.08]
lb = [0.0, 0.0, 0.0]
pkac1_fit = curve_fit(model, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pkac1_coef = coef(pkac1_fit)

3-element Vector{Float64}:
 0.19937289218804352
 0.013906164279704621
 0.07340270820308735

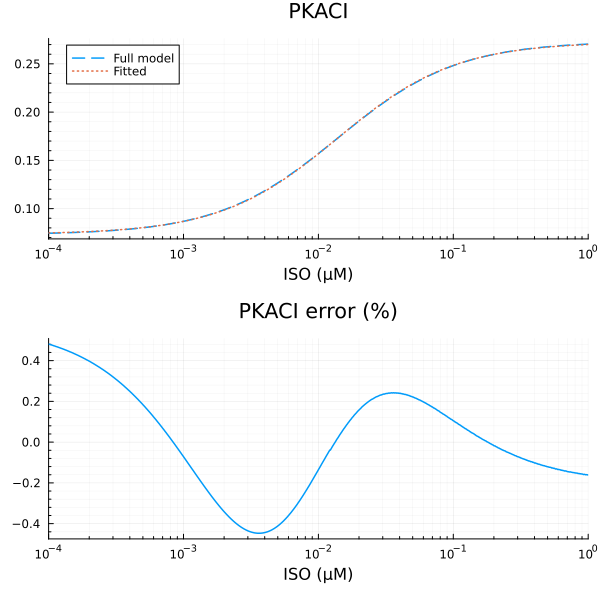
println("PKACI")
println("Basal activity: ", pkac1_coef[3])
println("Activated activity: ", pkac1_coef[1])
println("Michaelis constant: ", pkac1_coef[2], " μM")
println("RMSE: ", rmse(pkac1_fit))

PKACI
Basal activity: 0.07340270820308735
Activated activity: 0.19937289218804352
Michaelis constant: 0.013906164279704621 μM
RMSE: 0.0003269286290755473

ypred = model.(xdata, Ref(pkac1_coef))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="PKACI", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="PKACI error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

Fitting active PKACII

xdata = iso
ydata = extract(sim, sys.PKACII / sys.RIItot)
p0 = [0.4, 0.01μM, 0.2]
lb = [0.0, 0.0, 0.0]
pkac2_fit = curve_fit(model, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pkac2_coef = coef(pkac2_fit)

3-element Vector{Float64}:
 0.3443707303688551
 0.010251017585274896
 0.18399253616222164

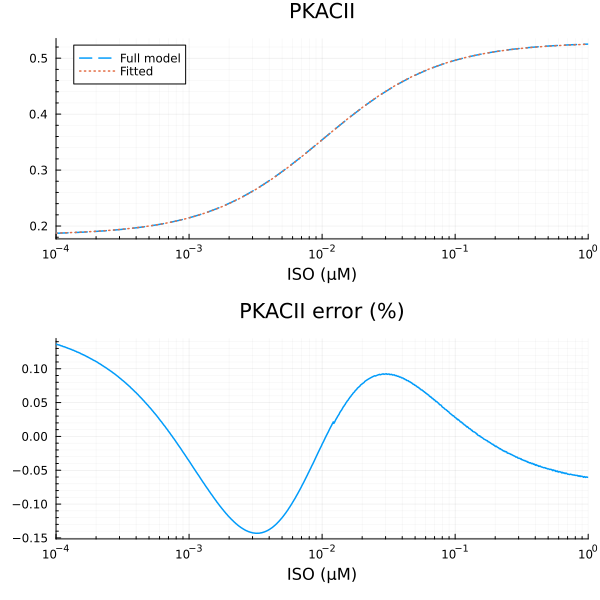
println("PKACII")
println("Basal activity: ", pkac2_coef[3])
println("Activated activity: ", pkac2_coef[1])
println("Michaelis constant: ", pkac2_coef[2], " μM")
println("RMSE: ", rmse(pkac2_fit))

PKACII
Basal activity: 0.18399253616222164
Activated activity: 0.3443707303688551
Michaelis constant: 0.010251017585274896 μM
RMSE: 0.00024370430856219033

ypred = model.(xdata, Ref(pkac2_coef))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="PKACII", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="PKACII error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

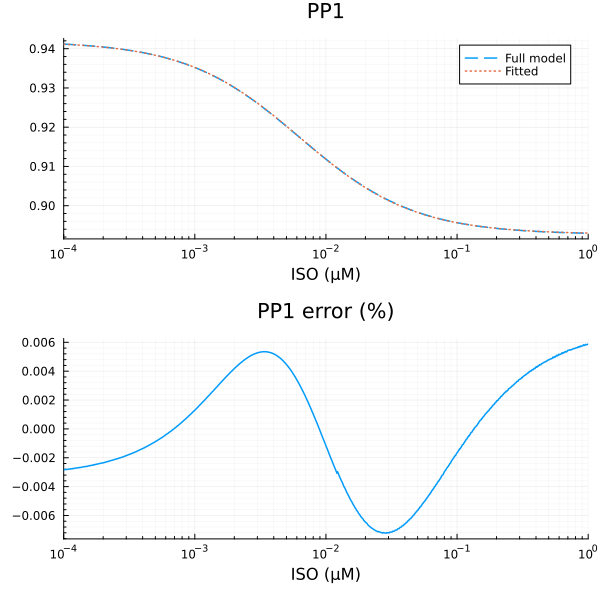
Least-square fitting of PP1 activity

@. model_pp1(x, p) = p[1] * p[2] / (x + p[2]) + p[3]
xdata = iso
ydata = extract(sim, sys.PP1 / sys.PP1totBA)
p0 = [0.1, 3e-3μM, 0.8]
lb = [0.0, 0.0, 0.0]
pp1_fit = curve_fit(model_pp1, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pp1_coef = coef(pp1_fit)

3-element Vector{Float64}:
 0.049191949706500135
 0.006361894471202522
 0.8927131245434415

println("PP1")
println("Repressible activity: ", pp1_coef[1])
println("Minimal activity: ", pp1_coef[3])
println("Repressive Michaelis constant: ", pp1_coef[2], " μM")
println("RMSE: ", rmse(pp1_fit))

PP1
Repressible activity: 0.049191949706500135
Minimal activity: 0.8927131245434415
Repressive Michaelis constant: 0.006361894471202522 μM
RMSE: 3.5404185424829945e-5

ypred = model_pp1.(xdata, Ref(pp1_coef))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="PP1", legend=:topright; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="PP1 error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

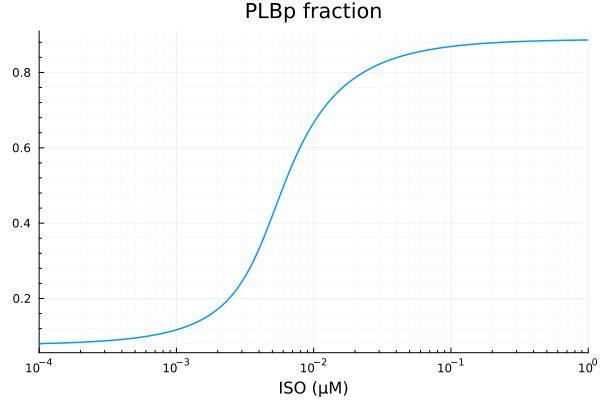
Fitting PLBp

xdata = iso
ydata = extract(sim, sys.PLBp / sys.PLBtotBA)
plot(xdata, ydata, title="PLBp fraction", lab=false; xopts...)

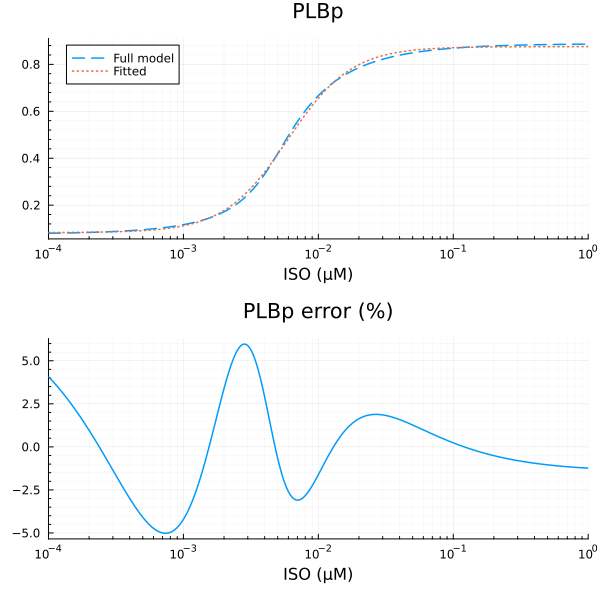
First try: Hill function

@. model_plb(x, p) = p[1] * hil(x, p[2], p[3]) + p[4]
p0 = [0.8, 1e-2μM, 1.0, 0.1]
lb = [0.5, 1e-9μM, 1.0, 0.0]
fit = curve_fit(model_plb, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pestim = coef(fit)

4-element Vector{Float64}:
 0.7923203200989488
 0.005936642749285824
 1.8386686433022672
 0.08301223024865272

println("PLBp")
println("Basal activity: ", pestim[4])
println("Activated activity: ", pestim[1])
println("Michaelis constant: ", pestim[2], " μM")
println("Hill coefficient: ", pestim[3])
println("RMSE: ", rmse(fit))

PLBp
Basal activity: 0.08301223024865272
Activated activity: 0.7923203200989488
Michaelis constant: 0.005936642749285824 μM
Hill coefficient: 1.8386686433022672
RMSE: 0.008419217103593078

ypred = model_plb.(xdata, Ref(pestim))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="PLBp", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="PLBp error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

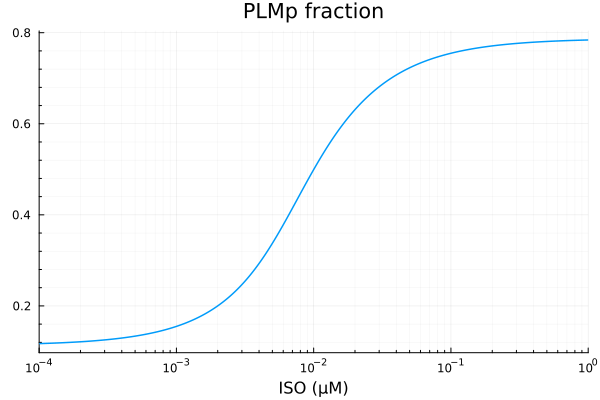
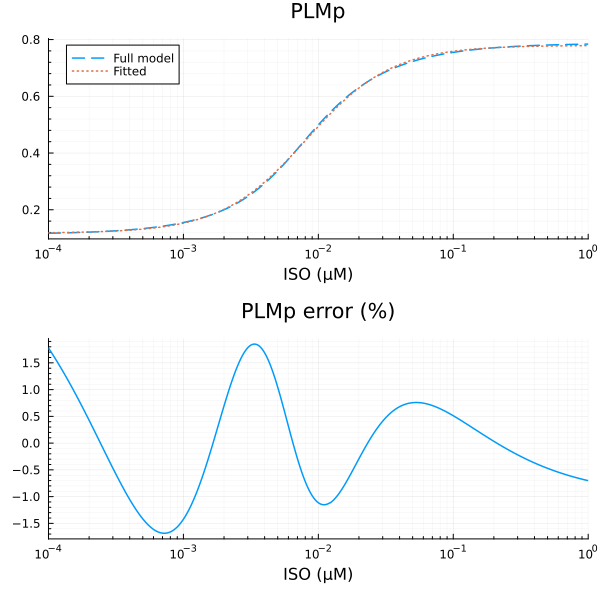
Fitting PLMp

xdata = iso
ydata = extract(sim, sys.PLMp / sys.PLMtotBA)
plot(xdata, ydata, title="PLMp fraction", lab=false; xopts...)

@. model_plm(x, p) = p[1] * hil(x, p[2], p[3]) + p[4]
p0 = [0.8, 1e-2μM, 1.0, 0.1]
lb = [0.5, 1e-9μM, 1.0, 0.0]
fit = curve_fit(model_plm, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pestim = coef(fit)

4-element Vector{Float64}:
 0.6617188859975476
 0.008181708846395365
 1.371034816343858
 0.11772725193948856

println("PLMp")
println("Basal activity: ", pestim[4])
println("Activated activity: ", pestim[1])
println("Michaelis constant: ", pestim[2], " μM")
println("Hill coefficient: ", pestim[3])
println("RMSE: ", rmse(fit))

PLMp
Basal activity: 0.11772725193948856
Activated activity: 0.6617188859975476
Michaelis constant: 0.008181708846395365 μM
Hill coefficient: 1.371034816343858
RMSE: 0.0032738776162412253

ypred = model_plm.(xdata, Ref(pestim))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="PLMp", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="PLMp error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

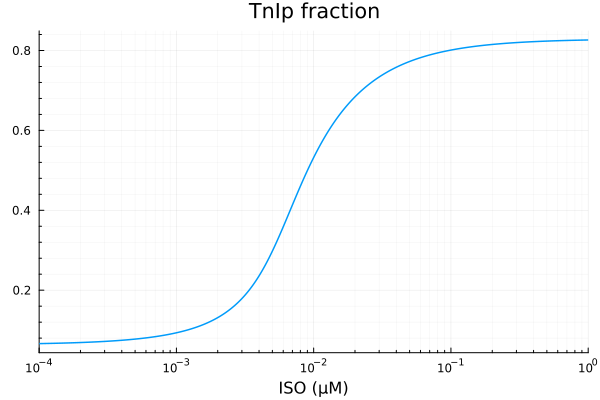
Fitting TnIp

xdata = iso
ydata = extract(sim, sys.TnIp / sys.TnItotBA)
plot(xdata, ydata, title="TnIp fraction", lab=false; xopts...)

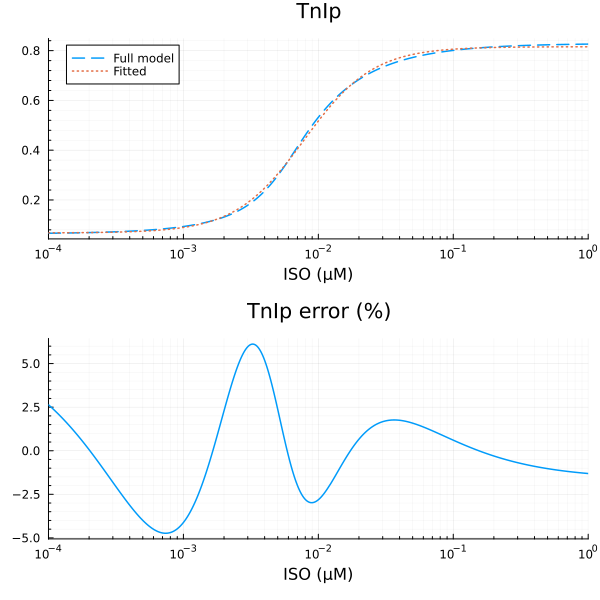
@. model_tni(x, p) = p[1] * hil(x, p[2], p[3]) + p[4]
p0 = [0.8, 1e-2μM, 1.0, 0.1]
lb = [0.1, 1e-9μM, 1.0, 0.0]
fit = curve_fit(model_tni, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pestim = coef(fit)

4-element Vector{Float64}:
 0.7481954356008236
 0.007856336940166107
 1.6973368393770933
 0.06752961149817202

println("TnIp")
println("Basal activity: ", pestim[4])
println("Activated activity: ", pestim[1])
println("Michaelis constant: ", pestim[2], " μM")
println("Hill coefficient: ", pestim[3])
println("RMSE: ", rmse(fit))

TnIp
Basal activity: 0.06752961149817202
Activated activity: 0.7481954356008236
Michaelis constant: 0.007856336940166107 μM
Hill coefficient: 1.6973368393770933
RMSE: 0.0074371506078312385

ypred = model_tni.(xdata, Ref(pestim))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="TnIp", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="TnIp error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

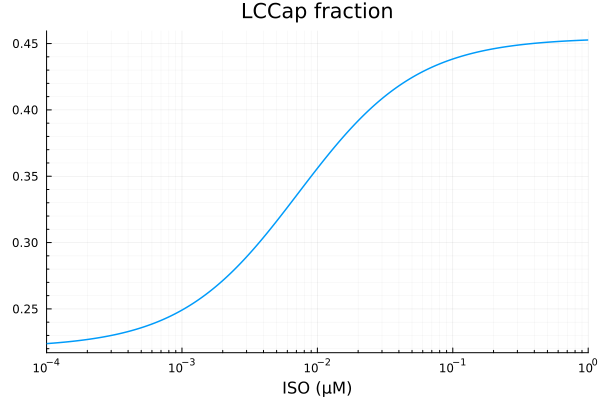
Fitting LCCap

xdata = iso
ydata = extract(sim, sys.LCCap / sys.LCCtotBA)
plot(xdata, ydata, title="LCCap fraction", lab=false; xopts...)

@. model_lcc(x, p) = p[1] * hil(x, p[2]) + p[3]
p0 = [0.8, 1e-2μM, 0.1]
lb = [0.1, 1e-9μM, 0.0]
fit = curve_fit(model_lcc, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pestim = coef(fit)

3-element Vector{Float64}:
 0.23338201681192608
 0.007263349265270028
 0.22081945564673972

println("LCCap")
println("Basal activity: ", pestim[3])
println("Activated activity: ", pestim[1])
println("Michaelis constant: ", pestim[2], " μM")
println("RMSE: ", rmse(fit))

LCCap
Basal activity: 0.22081945564673972
Activated activity: 0.23338201681192608
Michaelis constant: 0.007263349265270028 μM
RMSE: 0.00013472080219164504

ypred = model_lcc.(xdata, Ref(pestim))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="LCCap", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="LCCap error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

Fitting LCCbp

xdata = iso
ydata = extract(sim, sys.LCCbp / sys.LCCtotBA)
plot(xdata, ydata, title="LCCbp fraction", lab=false; xopts...)

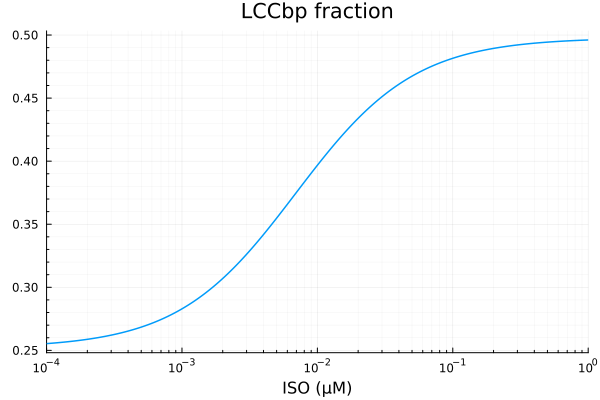
p0 = [0.8, 1e-2μM, 0.1]
lb = [0.1, 1e-9μM, 0.0]
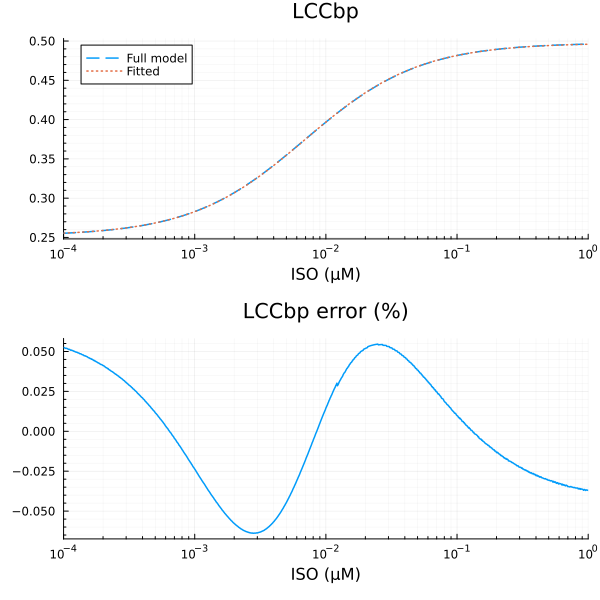
fit = curve_fit(model_lcc, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pestim = coef(fit)

3-element Vector{Float64}:
 0.2455890136661459
 0.006961124585982423
 0.2520079822710803

println("LCCbp")
println("Basal activity: ", pestim[3])
println("Activated activity: ", pestim[1])
println("Michaelis constant: ", pestim[2], " μM")
println("RMSE: ", rmse(fit))

LCCbp
Basal activity: 0.2520079822710803
Activated activity: 0.2455890136661459
Michaelis constant: 0.006961124585982423 μM
RMSE: 0.000138054179142439

ypred = model_lcc.(xdata, Ref(pestim))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="LCCbp", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="LCCbp error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

Fitting KURp

xdata = iso
ydata = extract(sim, sys.KURp / sys.IKurtotBA)
plot(xdata, ydata, title="LCCbp fraction", lab=false; xopts...)

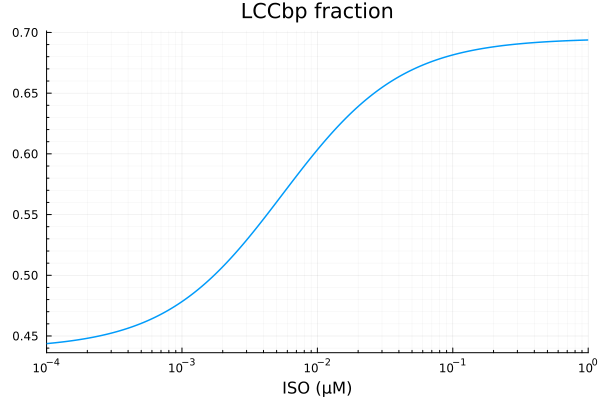
@. model_kur(x, p) = p[1] * hil(x, p[2]) + p[3]
p0 = [0.8, 1e-2μM, 0.1]
lb = [0.1, 1e-9μM, 0.0]
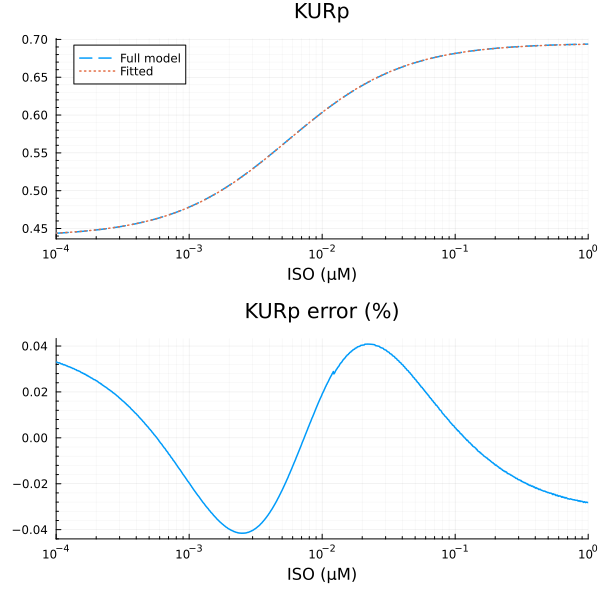
fit = curve_fit(model_kur, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pestim = coef(fit)

3-element Vector{Float64}:
 0.25565723778714106
 0.005578155557974466
 0.4393640045672578

println("KURp")
println("Basal activity: ", pestim[3])
println("Activated activity: ", pestim[1])
println("Michaelis constant: ", pestim[2], " μM")
println("RMSE: ", rmse(fit))

KURp
Basal activity: 0.4393640045672578
Activated activity: 0.25565723778714106
Michaelis constant: 0.005578155557974466 μM
RMSE: 0.00014848030234696259

ypred = model_lcc.(xdata, Ref(pestim))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="KURp", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="KURp error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

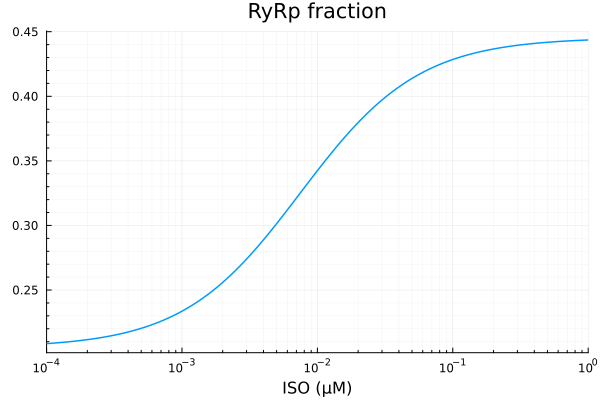
Fitting RyRp

xdata = iso
ydata = extract(sim, sys.RyR_PKAp)
plot(xdata, ydata, title="RyRp fraction", lab=false; xopts...)

@. model(x, p) = p[1] * x / (x + p[2]) + p[3]
p0 = [0.3, 1e-2μM, 0.1]
lb = [0.0, 1e-9μM, 0.0]
fit = curve_fit(model, xdata, ydata, p0; lower=lb, autodiff=:forwarddiff)
pestim = coef(fit)

3-element Vector{Float64}:
 0.2398866934142532
 0.007509869883108145
 0.20539803429132422

println("RyRp")
println("Basal activity: ", pestim[3])
println("Activated activity: ", pestim[1])
println("Michaelis constant: ", pestim[2], " μM")
println("RMSE: ", rmse(fit))

RyRp
Basal activity: 0.20539803429132422
Activated activity: 0.2398866934142532
Michaelis constant: 0.007509869883108145 μM
RMSE: 7.686343949045511e-5

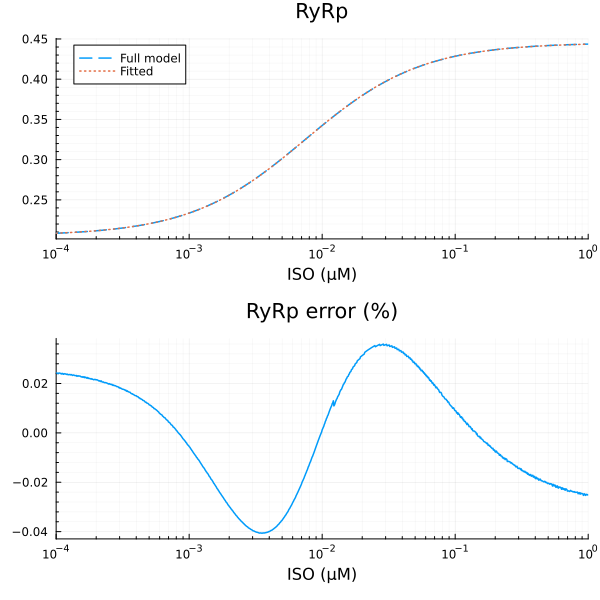
ypred = model.(xdata, Ref(pestim))
p1 = plot(xdata, [ydata ypred], lab=["Full model" "Fitted"], line=[:dash :dot], title="RyRp", legend=:topleft; xopts...)
p2 = plot(xdata, (ypred .- ydata) ./ ydata .* 100; title="RyRp error (%)", lab=false, xopts...)
plot(p1, p2, layout=(2, 1), size=(600, 600))

---

This notebook was generated using Literate.jl.